Escher-like Spiral Tilings
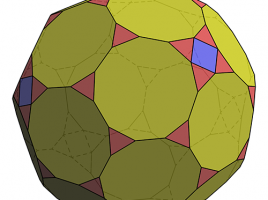
A Molecular Near Miss!
Hexagonal Cross Stitch
At least year’s Bridges Conference in Stockholm, I attended a short presentation by Susan Goldstine about “self-diagramming lace”. As motivation for the new work she was presenting, Susan referenced her paper from the year before on what she calls “symmetry samplers”. Samplers are an old tradition in fibre arts. A …
The Tactile libraries
I developed a new open-source software library for manipulating isohedral tilings, based on the work I did on this topic during my PhD. The library is available in C++ and Javascript, and I offer a few fun automated and interactive demo programs that anybody can use to play with isohedral tilings.
Tilted Espresso Cup
Here’s the second of two new espresso cups (read about the other one). The concept is simple: slice through the bottom of the cup and the handle at an angle, so that the cup looks like it’s sinking into the table. I think I can say with some confidence that …
Moka Pot Espresso Cup
During the holidays at the end of 2017, I had a bit of time to return to my occasional hobby of 3D printing. I figured I would design a couple of new espresso cups. I always seem to come back to 3D printed porcelain cups. I suppose it’s a nice …
Heesch Numbers, Part 4: Edge-to-Edge Pentagons
This post is the fourth and final one in a series about Heesch numbers. Part 1 was a general introduction, and would be a good starting point if you’re unfamiliar with the topic. Part 2 covered exhaustive computations of Heesch numbers of polyominoes and polyiamonds, and likely isn’t needed to …
Heesch Numbers, Part 3: Bamboo Shoots and Ice Cream Cones
This is the third post in a planned series of four about Heesch numbers. In the first post, I introduced some of the basic ideas behind Heesch numbers; if you’re not familiar with the topic, you may want to read it before coming back here. The second post was about …
Heesch Numbers, Part 2: Polyforms
In the first post in this series, I introduced the concept of a shape’s Heesch number. In brief, if a shape doesn’t tile the plane, its Heesch number is a measure of the maximum number of times you can surround the shape with layers of copies of itself. (Shapes that do …